Cardinal utility analysis attempts to explain the logic behind consumer behaviour by attaching value to the utility derived from the consumption of a commodity. By consuming a commodity that individuals want, they derive some utility or satisfaction from it. According to cardinal utility, this satisfaction derived from the consumption of a good can be measured (generally measured and referred to as utils). Therefore, consumers make their consumption choices based on that measured utility and choose the quantity of every commodity to consume in such a manner that it maximizes their satisfaction.
The utility can, therefore, be referred to as the want-satisfying power of a commodity. The concept of utility involves two types of measures:
- Total utility: it is the total amount of utility that a consumer derives by consuming a specific quantity of a commodity or service. For example, total utility derived from consuming 5 cupcakes.
- Marginal utility: it is the additional satisfaction that a consumer gets by consuming an additional unit of a commodity or service. For example, it’s the extra utility that a consumer gets from eating the 6th cupcake. It can also be defined as the change in total utility resulting from one more unit of commodity consumed.
Econometrics Tutorials with Certificates
Law of Diminishing Marginal Utility
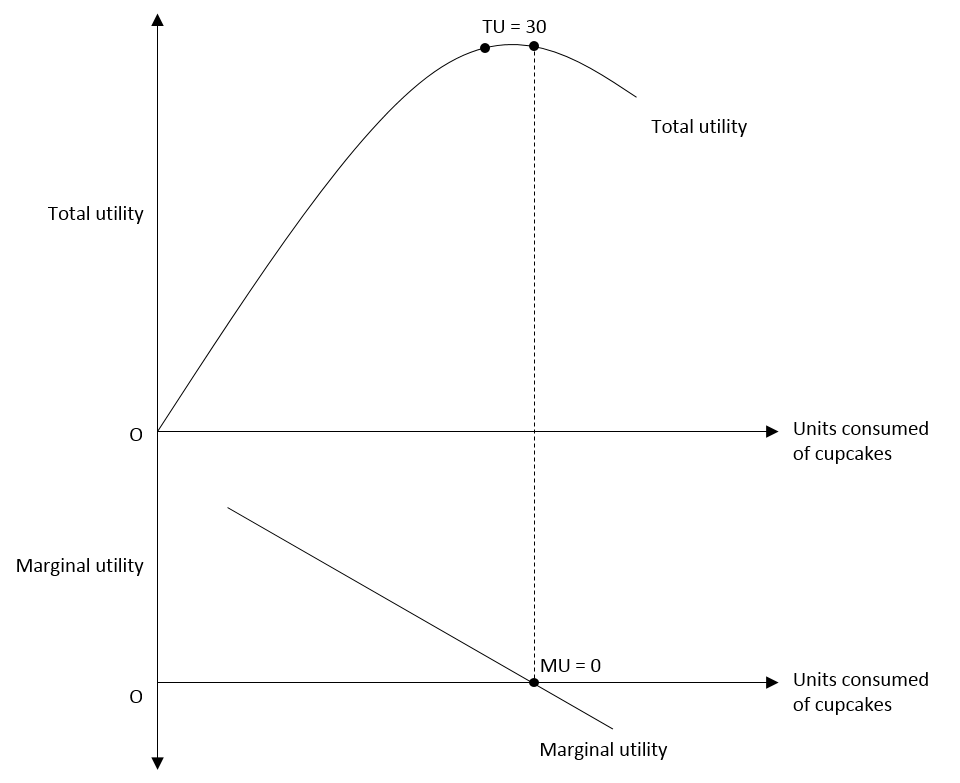
The Law of diminishing marginal utility is the foundation of cardinal utility analysis. This law states that the marginal utility derived from the consumption of each unit of a good or service goes on declining as more quantity is consumed. In other words, the extra satisfaction from consumption of each successive unit of a commodity keeps falling.
For example, as the consumer keeps eating more cupcakes, satisfaction from each additional cupcake will keep declining, i.e. additional satisfaction from 2nd cupcake will be high as compared to 3rd cupcake. Similarly, additional satisfaction from the 3rd cupcake will be higher than that from the 4th cupcake and so on. Eventually, marginal utility or additional satisfaction will become negative and total utility will start falling.
| Units of cupcakes consumed | Total utility (utils) | Marginal utility (utils) |
| 0 | 0 | 0 |
| 1 | 10 | 10 |
| 2 | 18 | 8 |
| 3 | 24 | 6 |
| 4 | 28 | 4 |
| 5 | 30 | 2 |
| 6 | 30 | 0 |
| 7 | 28 | -2 |
As more cupcakes are consumed, marginal utility from each unit keeps decreasing, falls to zero and becomes negative. It is also reflected in the total utility, where, total utility keeps increasing till marginal utility is positive. This increase in total utility is at a decreasing rate because the marginal utility is falling. Total utility is maximum when marginal utility is zero and it starts decreasing when marginal utility becomes negative.
Slope of Demand Curve
The Law of diminishing marginal utility also explains why demand curves are generally downward sloping. According to this law, marginal utility decreases with each successive unit. Therefore, consumers will buy an additional unit of a commodity only at a lower price. Since consuming a good further gives lesser marginal utility, consumers will be willing to pay a lesser price for the good or service.
Hence, demand curves are downward sloping because more of a commodity or service is purchased only at a lower price. Consumers will choose to buy other goods that give higher utility if the price of the commodity remains high.
Consumer Equilibrium under Cardinal Utility Analysis (Utility Maximization)
Given a number of goods, consumers will purchase a combination of those goods in such a manner so as to maximize their utility or satisfaction. In order to maximize utility, consumers spend their income on each good in a way that the last dollar (or any currency) spent on each good gives the same amount of marginal utility. At this point, the consumer will be at equilibrium. There will be no incentive to change the purchase pattern. This can also be represented as follows:
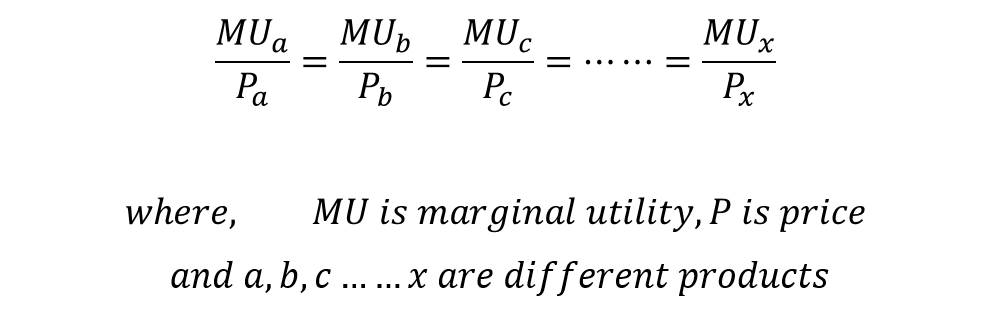
Given the budget, the utility will be maximum when the ratios of marginal utility (MU) to price (P) of all the goods are equal. The quantity of each good to be purchased will be decided by the equivalence of these ratios.
Example of Cardinal Utility Analysis
Let us consider an example with 2 goods- cupcakes and muffins. Suppose the budget or income of the consumer is 16 dollars, the price of cupcakes is 2 dollars and the price of muffins is 4 dollars.
| Units of commodity | MU of cupcakes (utils) | MU/P of cupcakes | MU of muffins (utils) | MU/P of muffins |
| 1 | 20 | 10 | 30 | 7.5 |
| 2 | 18 | 9 | 28 | 7 |
| 3 | 16 | 8 | 26 | 6.5 |
| 4 | 14 | 7 | 22 | 5.5 |
| 5 | 12 | 6 | 18 | 4.5 |
| 6 | 10 | 5 | 14 | 3.5 |
| 7 | 8 | 4 | 10 | 2.5 |
Therefore, consumer will purchase 4 units of cupcakes and 2 units of muffins with 16 dollars of budget, where:
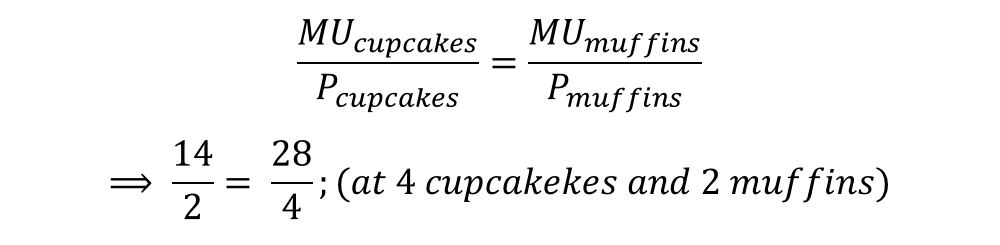
The ratio of marginal utility to the price of that commodity implies that consumers consider both, the benefit and the costs of purchasing additional units of a product. Marginal utility represents the benefits and the price of good represents the cost that the consumer has to pay for that extra utility.
Hence, consumer equilibrium is achieved with a balance between the benefit and cost of every product. At equilibrium, consumers will have no incentive to change expenditure pattern because the utility is already at maximum.
Assumptions of Cardinal Utility Analysis
- Consumers are rational, i.e. their aim is to maximize utility.
- Utility can be measured (in units or values called utils)
- Marginal utility of money remains constant
- Price of one good does not affect the marginal utility of the other.
- Tastes and preference of consumer remain constant.
Diamond-Water Paradox
Earlier economists were confused by the fact that water, which is essential for survival, has such low prices. Whereas, diamonds are expensive even though they are not essential goods. This paradox can be explained with this cardinal utility analysis and utility maximization.
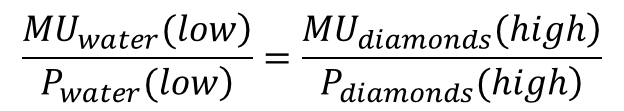
The price of water is low because its supply is abundant relative to its demand. On the other hand, diamonds are a rare commodity and their supply is meagre relative to its demand. Hence, diamonds have high prices as compared to water. Since water is priced low, it is used for numerous purposes. With more and more units consumed, the marginal utility of an extra litre of water is also low because its marginal utility keeps falling with high usage.
Conversely, diamonds have high prices leading to fewer purchases which keep their marginal utility high. As a result, the ratio of marginal utility to the price of both water and diamond end up being similar. Consumers, therefore, purchase diamonds even though they are not essential.
Note: total utility of water is much higher than diamonds because water is used for numerous purposes and consumed a lot more. That is, water still holds its usefulness because the total utility is a sum of all marginal utilities. Diamonds, however, have low total utility because they are not essential and consumed less. It is important to understand here that marginal utility is considered for additional consumption and not total utility.
Criticism
The major drawback of cardinal utility analysis is its assumption that utility can be measured accurately. It is unrealistic to assume that consumers can assign an accurate value to something as subjective as satisfaction or utility. It is impossible to quantify and assign numerical values to utility in terms of utils, which does not make sense.
However, it is possible to rank preferences based on utility, i.e. to recognize which product gives more utility. Ordinal utility analysis was, hence, developed which focuses on ordering preferences instead of measuring. The logic of ordinal utility makes more sense because consumers know what they prefer. But it is impossible to assign value to measure by “how much” they prefer a good or service.
Econometrics Tutorials with Certificates
This website contains affiliate links. When you make a purchase through these links, we may earn a commission at no additional cost to you.



goodwork
Thank you!!
help me with the approach for that question
Please let me know more about the question that you want to discuss. I ll be happy to help
discuss different types of cobb doghlous production function
Hello. For production functions and the Cobb Douglas Production Function, you can refer to the following posts:
1) https://spureconomics.com/cobb-douglas-production-function/
2) https://spureconomics.com/production-function-definition-and-types/
the way you explained every point is so simple to understand. my vague concepts are now crystal clear because of your explanation.. thank you 🙏
You’re welcome and thank you for your appreciation 🙂