The Goldfeld Quandt Test is used to detect the presence of heteroscedasticity in a regression model. This test was introduced by economists Arthur Goldfeld and Richard Quandt in the 1960s. It involves dividing the data further into sub-groups and analysing the residual variances of the groups. Hence, the residual variances of the groups will be significantly different if there is heteroscedasticity.
Moreover, the sub-groups are decided based on the values of an independent variable. If there is more than one independent variable in the model, we must choose any one of them to decide the sub-groups. This test is often used in conjunction with other heteroscedasticity tests such as the White test or the Breusch Pagan Test.
Econometrics Tutorials with Certificates
Application of the Goldfeld Quandt Test
Step 1: Firstly, order the observations according to the values of the independent variable (Xi) in ascending order. If there are multiple independent variables, one of them must be chosen to order the observations. You can also select the independent variable that is suspected of being related to the problem of heteroscedasticity.
Step 2: Choose ‘c’, the number of central observations that need to be omitted from the sub-groups. We must divide the remaining observations into 2 groups- one with the first and the other with the last observations. The first group contains observations smaller than the central observations. Therefore, the last group contains observations larger than the central or omitted observations.
Step 3: then, we run separate regressions on both sub-groups. Furthermore, the independent variables will be the same for both regressions.
Step 4: calculate the residual sum of squares for both regressions RSS1 and RSS2, corresponding to the smaller and larger observation groups respectively.
Step 5: compute the following:
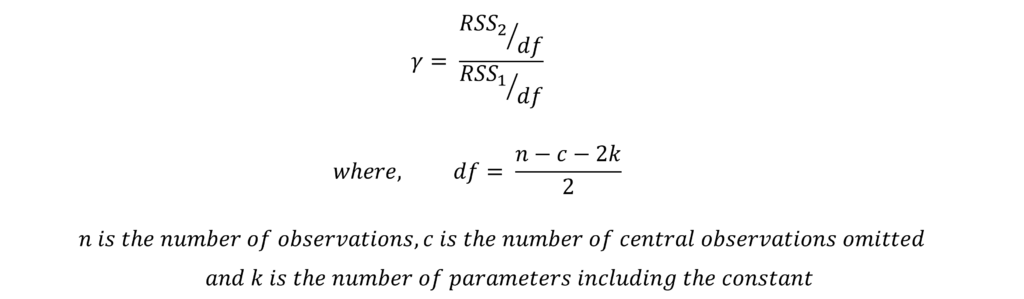
The variable γ follows the F-distribution with the degree of freedom defined above. If this value is greater than the critical F-value at the given level of significance, we reject the null hypothesis of homoscedasticity. Hence, we conclude that heteroscedasticity exists.
Limitations of the test
Finally, there are some limitations associated with the test that must be considered:
- The ‘c’ or central observations to be omitted must be carefully chosen. This is because a wrong choice may lead to unreliable results.
- For multiple independent variables, it becomes difficult to know beforehand which variable should be chosen to order the observations. Separate testing is required to determine which variable is appropriate.
This test is preferred over the Park test and Glejser’s test. Goldfeld and Quandt suggested that c = 8 and c = 16 are usually appropriate for around n = 30 and n = 60 observations respectively.
Econometrics Tutorials with Certificates
This website contains affiliate links. When you make a purchase through these links, we may earn a commission at no additional cost to you.


